Starting up...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Calculus BC

Calculus BC Parametric Differentiation

Section 2: Differentiation: Lecture 1 | 13:15 min

Lecture Description

Next Lecture

Previous Lecture

Discussion
Answer Engine
Study Guides
Practice Questions
Download Lecture Slides
Table of Contents
Related Books

Lecture Comments (9)

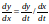
	0 answers Post by David Chen on March 13, 2018 For the 4th example, you substituted 2 for t into your derivative, but at -2t^2, you added 2*2^2. Was that a mistake or am I missing something here?
	2 answers Last reply by: Patricia Xiang Thu May 3, 2018 2:21 AM Post by Luvivia Chang on April 1, 2015 In example 3, the question is asking for a tangent line, not necessary a tangent line that is horizontal. So the line should be an equation with variable t, not an exact point.
	1 answer Last reply by: Craig McRae Wed Jul 31, 2013 2:51 PM Post by chetachi ohagi on June 14, 2013 Where did he get the y prime derivative from in example 2?
	2 answers Last reply by: Luvivia Chang Wed Apr 1, 2015 7:30 AM Post by Christopher Lagos on May 3, 2012 Why is it that when solving for the acceleration you leave it as a coordinate rather than a single quantity.

Answer EngineGet answers to any question!Ask any question related to Calculus BC

Working on the solution...

Parametric Differentiation

Differentiate the 2 parametric parts separately
Divide
Simplify

Parametric Differentiation

A parametric curve is defined by:
x(t) = 3t − 1
y(t) = 7 − 5t
Find [dy/dx]

Find [dx/dt]
x(t) = 3t − 1
x′(t) = 3
Find [dy/dt]
y(t) = 7 − 5t
y′(t) = − 5

Divide y′(t) by x′(t) to find [dy/dx][dy/dx] = [( − 5)/3]

A parametric curve is defined by:
x(t) = [2/t] − 2
y(t) = [1/((t − 1)²)]
Find [dy/dx]

Find [dx/dt]
x(t) = [2/t] − 2
x′(t) = − 2(t)^{− 2}
Find [dy/dt]
y(t) = [1/((t − 1)²)]
y′(t) = − (t − 1)^{− 2}
Divide y′(t) by x′(t) to find [dy/dx]
[dy/dx] = [( − (t − 1)^{− 2})/( − 2(t)^{− 2})]

= [(2t²)/((t − 1)²)]

A parametric curve is defined by:
x(t) = 5t + 2
y(t) = √{6t}
Find [dy/dx], for t = 3

Find [dx/dt]
x(t) = 5t + 2
x′(t) = 5
Find [dy/dt]
y(t) = √{6t}
y′(t) = [1/(6√{6t} )]
Divide y′(t) by x′(t) to find [dy/dx]
[dy/dx] = [([1/(6√{6t} )])/5]
= [1/(30√{6t} )]

Solve for t = 3[1/(30√{6t} )] = [1/(30√{6(3)} )] = [1/(30√{18} )]

A parametric curve is defined by:
y(t) = t² + 8t − 3
x(t) = cos([t/2] − 1)
Find [dy/dx] for t = 4

Find [dx/dt]
x(t) = cos([t/2] − 1)
x′(t) = − 2sin([t/2] − 1)
Find [dy/dt]
y(t) = t² + 8t − 3
y′(t) = 2t + 8
Divide y′(t) by x′(t) to find [dy/dx]
[dy/dx] = [(2t + 8)/( − 2sin([t/2] − 1))]
= [(t + 4)/( − sin([t/2] − 1))]
Solve for t = 4
[(t + 4)/( − sin([t/2] − 1))] = [(4 + 4)/( − sin([4/2] − 1))]
= [8/( − sin(1))]

= −9.51 (in rads)

If a function is defined parametrically as (3t^3,,t² − 2t + 8), what is its [dy/dx]?

Find [dx/dt]
x(t) = 3t^3,
x′(t) = 9t²
Find [dy/dt]
y(t) = t² − 2t + 8
y′(t) = 2t − 2

Divide y′(t) by x′(t) to find [dy/dx][dy/dx] = [(2t − 2)/(9t²)]

If a particle's position is defined parametrically as (3t^3,,t² − 2t + 8), what is it's speed at t = 6?

Use the definition of speed
speed = √{([dx/dt])² + ([dy/dt])²}
= √{(9t²)² + (2t − 2)²}

Solve for t = 6√{(9t²)² + (2t − 2)²} = √{(9(6)²)² + (2(6) − 2)²} = 20.59

If a particle's position is defined parametrically as (3t^3,,t² − 2t + 8), what is it's acceleration at t = 3?

Find [(d²x)/(dt²)]
[dx/dt] = 9t²
[(d²x)/(dt²)] = 18t
Find [(d²y)/(dt²)]
[dy/dt] = 2t − 2
[(d²y)/(dt²)] = 2
Use the definition of acceleration
acceleration = < [(d²y)/(dt²)],[(d²x)/(dt²)] >
= < 18t,2 >
Solve for t = 3
< 18t,2 > = < 18(3),2 >

= < 44,2 >

If a function is defined parametrically as ([(t²)/2],t² − 7t + 10), what is its [dy/dx]?

Find [dx/dt]
x(t) = [(t²)/2]
x′(t) = t
Find [dy/dt]
y(t) = t² − 7t + 10
y′(t) = 2t − 7

Divide y′(t) by x′(t) to find [dy/dx][dy/dx] = [(2t − 7)/t]

If a particle's position is defined parametrically as ([(t²)/2],t² − 7t + 10), what is it's speed at t = 2?

Use the definition of speed
speed = √{([dx/dt])² + ([dy/dt])²}
= √{(t)² + (2t − 7)²}
Solve for t = 2
√{(t)² + (2t − 7)²} = √{(2)² + (2(2) − 7)²}

=3.61

If a particle's position is defined parametrically as ([(t²)/2],t² − 7t + 10), what is it's acceleration at t = 3?

Find [(d²x)/(dt²)]
[dx/dt] = t
[(d²x)/(dt²)] = 0
Find [(d²y)/(dt²)]
[dy/dt] = 2t − 7
[(d²y)/(dt²)] = 2
Use the definition of acceleration
acceleration = < [(d²y)/(dt²)],[(d²x)/(dt²)] >
= < 0,2 >
Solve for t = 3
< 0,2 > = < 0,2 >

= < 0,2 >

« Previous Question | Next Question »

*These practice questions are only helpful when you work on them offline on a piece of paper and then use the solution steps function to check your answer.

Answer

Parametric Differentiation

Lecture Slides are screen-captured images of important points in the lecture. Students can download and print out these lecture slide images to do practice problems as well as take notes while watching the lecture.

Intro

Parametric Differentiation

Example 1

Example 2

Example 3

Example 4

Parametric Differentiation: Position, Speed & Acceleration

Example 5

Example 6

Intro 0:00
Parametric Differentiation 0:15
Example 1 1:16
Example 2 1:54
Example 3 3:15
Example 4 4:59
Parametric Differentiation: Position, Speed & Acceleration 7:45
Example 5 8:32
Example 6 10:37

Mathematics: AP Calculus BC

Section 1: Prerequisites
	Parametric Curves	10:10
	Polar Coordinates	14:54
	Vector Functions	12:05
Section 2: Differentiation
	Parametric Differentiation	13:15
	Polar Differentiation	10:14
	L'Hopital's Rule	6:43
Section 3: Integration
	Integration By Parts	19:04
	Integration By Partial Fractions	22:04
	Improper Integrals	19:01
Section 4: Applications of Integration
	Logistic Growth	19:16
	Arc Length for Parametric & Polar Curves	17:40
	Area for Parametric & Polar Curves	4:33
Section 5: Sequences, Series, & Approximations
	Definition & Convergence	7:10
	Geometric Series	10:59
	Harmonic & P Series	3:58
	Integral Test	11:31
	Comparison Test	7:47
	Limit Comparison Test	9:40
	Ratio Test	11:20
	Alternating Series	9:11
	Absolute Convergence	7:13
	Power Series Convergence	12:52
	Taylor Series	15:11
	Power Series Operations	16:40
	Lagrange Error	7:09
Section 6: Practice Test
	AP Calc BC Practice Test Section 1: Multi Choice Part 1	15:45
	AP Calc BC Practice Test Section 1: Multi Choice Part 2	21:38
	AP Calc BC Practice Test Section 1: Multi Choice Part 3	17:31
	AP Calc BC Practice Test Section 1: Multi Choice Part 4	14:17
	AP Calc BC Practice Test Section 1: Multi Choice Part 5	22:45
	AP Calc BC Practice Test Section 1: Free Response Part 1	9:55
	AP Calc BC Practice Test Section 1: Free Response Part 2	10:08
	AP Calc BC Practice Test Section 1: Free Response Part 3	13:58

Related Books

	Cracking the AP Calculus BC Exam, 2015 Edition Author: Princeton Review ISBN: 804124825 Publisher: Princeton Review Year: 2014 The book includes 3 full length practice tests with detailed explanations, a review of all the key concepts, and targeted strateeies to ace th exam for your highest score.
	Cliffs AP: Calculus AB & BC, 3rd Edition Authors: Dale W Johnson, Kerry J King ISBN: 764586831 Publisher: Cliffs Notes Year: 2001 The book has a topic-by topic breakdown and lots of problem approach suggestions for both free response and multiple choice calculus questions. The book also includes two full length tests.

Related Books

Name	Description	Link
BookRenter.com	BookRenter.com is simply the most reliable online textbook rental service.	Visit BookRenter.com
PhysicsForums.com Homework Help	Physics Forums is a scientific community for students looking for math & science help.	Visit PhysicsForums.com Homework Help

John Zhu

Parametric Differentiation

Slide Duration:

Table of Contents

Section 1: Prerequisites

Parametric Curves

10m 10s

Intro