Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of College Calculus: Level II
For more information, please see full course syllabus of College Calculus: Level II
College Calculus: Level II Trigonometric Substitutions
Lecture Description
In this video we are going to talk about Trigonometric Substitutions. There are three main equations that we have for trigonometric substitution. First we are going to list them. The idea is that if we see any one of these three forms in an integral, then we can do what is called a Trigonometric Substitution to convert out integral into a trigonometric integral. Then we can use some of the trigonometric techniques that we learned in the previous lecture to solve the integral. The best way to learn this is to do lots of examples, so we are going to do some of them.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine , substitute
, substitute 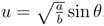 .
.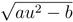 , substitute
, substitute 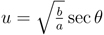 .
.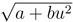 , substitute
, substitute 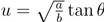 .
.





2 answers
Wed May 29, 2024 1:36 PM
Post by Nana kwame Afreh sika on May 18, 2024
why do i have trouble loading example 4 and example 5 videos
1 answer
Mon Feb 4, 2019 2:25 PM
Post by Hessa on February 1, 2019
how to solve this question using trig sub
the integral of x(1-x^4) ^1/2?
1 answer
Mon Jan 28, 2019 11:43 AM
Post by Hessa on January 27, 2019
I did not understand example 3
1 answer
Thu Aug 4, 2016 6:01 PM
Post by Peter Ke on July 27, 2016
For example 3, you let u = 3tan(theta). Where did that idea come from and why?
1 answer
Sat Feb 27, 2016 10:36 AM
Post by Mohsin Alibrahim on February 25, 2016
Dr Murray,
In example 3, how come du = [3sec^2(x)] while u = 9sec^2(x) ?
Thanks for the wonderful lecture
3 answers
Fri Apr 10, 2015 1:07 PM
Post by Luvivia Chang on April 7, 2015
Hello Dr. Murray, I have a question about exemple 1
i think the square root of (1-sin^2(theta))=the square root of cos^2(theta)= the "absolute value of cos(theta)" instead of just cos (theta)
Am I correct? Thanks
2 answers
Thu Feb 19, 2015 3:48 PM
Post by Thadeus McNamara on February 18, 2015
Dr. Murray, I am taking the Calc BC Exam. Are there any lectures in this College Calculus II playlist that I can skip because they are not a part of the exam? Thanks
2 answers
Thu Feb 19, 2015 3:48 PM
Post by Thadeus McNamara on February 18, 2015
@4:51, isn't the integral of 2/3sintheta equal to -2/3costheta? You put the integral as 2/3costheta.
3 answers
Wed Aug 27, 2014 9:46 AM
Post by brody wagerson on August 24, 2014
I believe there is an error in lecture example 4, on the last step to simplify the answer you cannot divide x^2-6x+9 by 9 to get x^2-6x, because they are addition and not multiplication. You must multiply out what is under the square root sign to get 9-(x^2-6x+9) the 9's will then cancel and give you 6x-x^2 under the square root just like in the beginning of the question, but without taking out an extra 1/3 to divide. Sorry if my explanation does not make sense I wrote this quickly. but i believe the correct answer to example 4 if I am correct will be 9/2[arcsin(x-3/3)+(x-3/3)*sqrt(6x-x^2)]+C. No stranger to criticism though so let me know if I made a mistake and professor Murray is right.
1 answer
Tue Aug 5, 2014 3:18 PM
Post by Pedro Valdericeda on July 21, 2014
Thank you Prof. Murray. You are really good.
1 answer
Tue Jun 17, 2014 12:03 PM
Post by ahmed ahmed on June 9, 2014
thank you professor! you are awesome!
1 answer
Mon Mar 17, 2014 1:13 PM
Post by Peidong, He on March 16, 2014
WANNA SAY THANK YOU!
1 answer
Fri Aug 23, 2013 11:52 AM
Post by Charles Zhou on August 21, 2013
This is absolutely a nice video! It does help me a lot. I just have a question. I'm a high school student and I wonder is there any difference or relationship between AP calculus BC and this College Calculus Level II
1 answer
Thu Dec 6, 2012 5:40 PM
Post by Samuel Bass on December 6, 2012
In problem #1 in the solution.
You converted cos(theta) = squar(1-sin^2(theta))
yes this would be correct if the cos(theta) was squared e.g. cos^2(theta)
but in this case the cos(theta) is not raised to the power 2.... then you can't use the pythagoream Identitie as you did in this case..... Im I correct? Cause I can't see this specific step you did possible. Please Explain.
1 answer
Mon Oct 22, 2012 1:48 PM
Post by Keisha Baxter on October 21, 2012
He factored incorrectly in Lecture example 1... in about the third line when he factors out the 4 from sqrt(4 - 4 sin^2 x) he puts 2 sqrt (1 - sin^2 x). So all of the coefficients following that is incorrect
1 answer
Thu Apr 18, 2013 12:24 PM
Post by SOUFIANE LAMOUNI on May 16, 2012
you are a great Professor , thank you
1 answer
Thu Apr 18, 2013 12:23 PM
Post by Kumar Sandrasegaran on February 26, 2012
Very very interesting. Makes difficult calculations look simple
1 answer
Thu Apr 18, 2013 12:20 PM
Post by Real Schiran on November 22, 2011
Very Nice, Thanks...........
1 answer
Thu Apr 18, 2013 12:19 PM
Post by Jaspreet Singh on February 21, 2010
this is so much more helpful than school! thanks for being so clear
1 answer
Thu Apr 18, 2013 12:19 PM
Post by lynette stevenson on February 17, 2010
excellent lecture