Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of College Calculus: Level I
For more information, please see full course syllabus of College Calculus: Level I
College Calculus: Level I Area Between Curves
Lecture Description
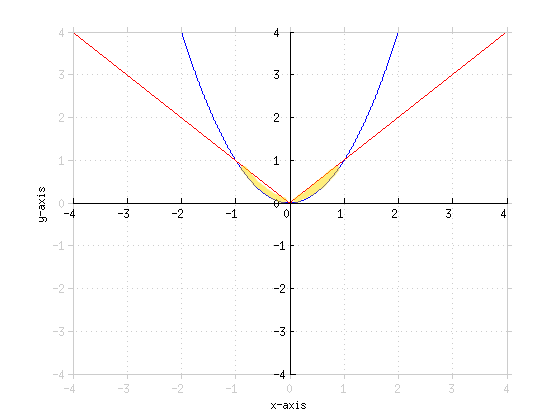
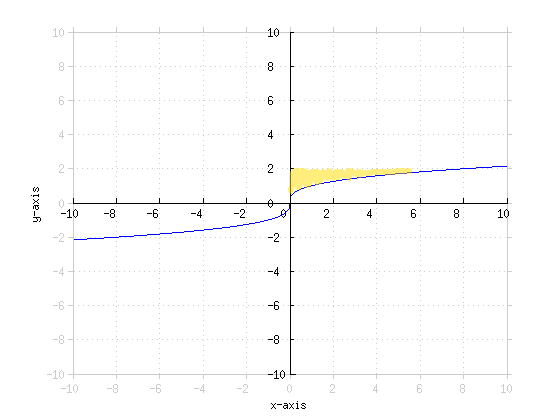
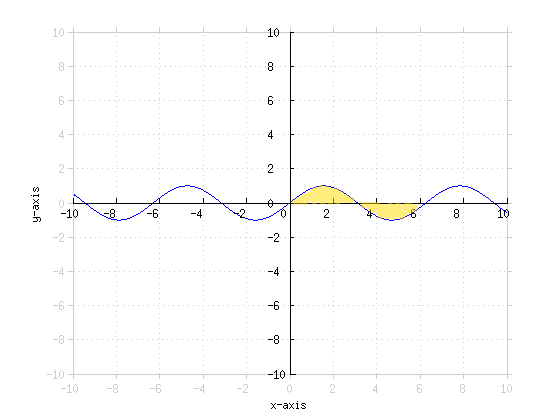
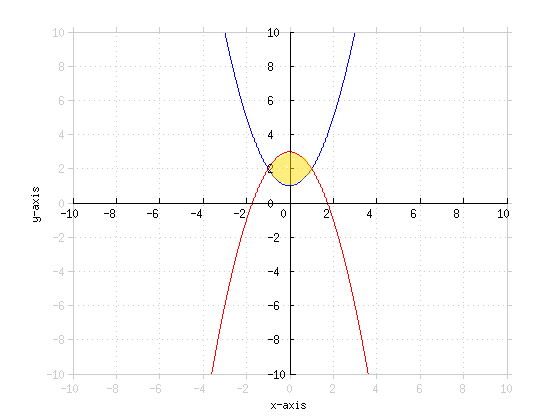
In this video we are going to look at finding the area between two curves using integration. First, we are going to introduce the integral for computing the area between two curves. We are going to see a rough sketch of what this would look like. To find the area between two curves we should first find out where the curves meet, which determines the endpoints of integration. Then we need to find an integrand which is the upper curve minus the lower curve. We will run through some examples to see how this runs in practice.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine







0 answers
Post by Eti Sinha on September 8, 2014
For additional example #5, is it possible to find the area underneath the curve by: Integral of ( 1/y ) dy, from 1/3 to 3? Using dy as opposed to dx?
0 answers
Post by Rufino Hernandez on July 5, 2014
Asides from getting the area of this triangle using this method, you can also get it using another method. The Area of a triangle is square root of (s(s-a)(s-b)(s-c)). Where s=(1/2)(a+b+c). Where a is one side of the triangle, b is another side of the triangle, and c is the third side of the triangle. Since the Triangle's lower angle is the same as the angle made by the function y=|x|, it can be deduced that the angle made is 90 degrees. If it is a 90 degree triangle, then we can simply use the Area of a triangle to come to the conclusion of the area; A=(1/2)b*h, where b=base and h=height. Since we have the end points of the sides of the triangle, we can simply derive sides. For example, the right side has an endpoint of (2,2); y=2, x=2. Using this we can make a second triangle where the sides are 2 and 2 which can lead us to find the third side which is square root of (8) = 2 * square root of(2). We now know the length of the right side of the triangle. Using this same same method, we can derive the length of the base of the triangle (bottom left). The endpoint of this side is (-2/3, -2/3). So we can make a third triangle that has these sides and derive the length of the second side of the original triangle. This second side will be (-2/3)^2+(-2/3)^2=c^2 ==> (4/9)+(4/9)= c^2 ==> 8/9=c^2 ==> c= (2 * square root of (2))/3. Now since we have the height and base, we can simply put this into the Area of a triangle; A=(1/2)(base)(height) ==> A=(1/2)((2square root of (2))/2)(2 * square root of (2) ==> 4/3. Or we can solve for the third side of the triangle and input them into the (The Area of a triangle is square root of (s(s-a)(s-b)(s-c)). Where s=(1/2)(a+b+c). Where a is one side of the triangle, b is another side of the triangle, and c is the third side of the triangle.) equation and get the same results. This method will yield the same answer as the method stated in this video.
0 answers
Post by Fadel Hanoun on December 17, 2013
What about when x = 5pi/4, where Tan = 1?
0 answers
Post by Troy Ling on February 4, 2011
k= -15/16
1 answer
Last reply by: Nopparat SAntisathaporn
Wed Oct 6, 2010 2:36 AM
Post by Nopparat SAntisathaporn on October 6, 2010
Hi there, I don't know how to do number 7 in this test http://www.nzqa.govt.nz/nqfdocs/ncea-resource/exams/2007/90286-exm-07.pdf
Can you please explain how to solve for K. Thank You.