Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Trigonometry
For more information, please see full course syllabus of Trigonometry
Trigonometry Law of Sines
Lecture Description
The Law of Sines is one of the really big rules of trigonometry and it tells us about the relationship between the sides and angles in any triangle (not just right triangles). We use the law of sines to solve triangles when we are given only some information about them, for example one side and two angles, or two sides and one angle. You'll see in the examples how to apply the sine rule to these triangles and you'll learn about the cases when there's one solution, two solutions or when there's no solution at all. You can master the sine rule by going through the examples.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine
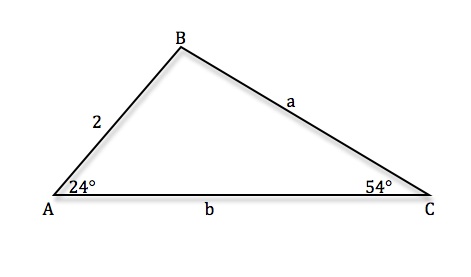
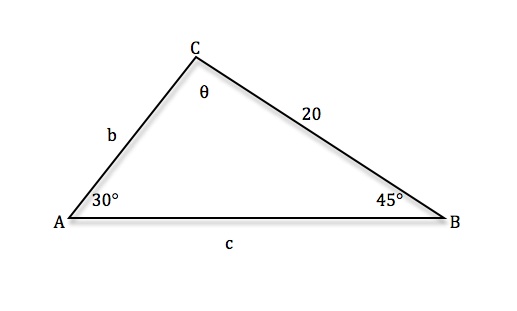
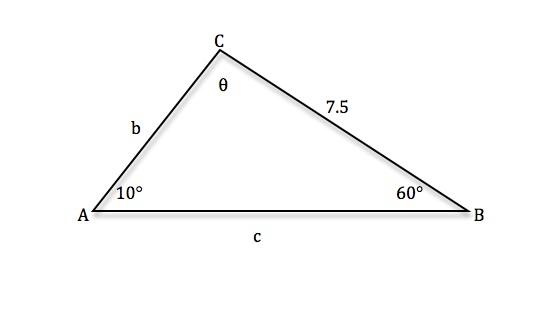
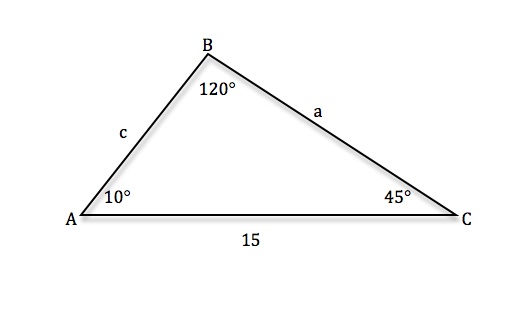
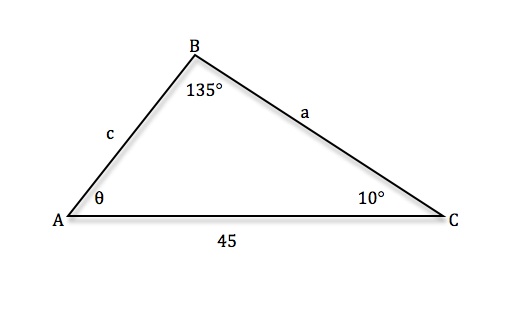
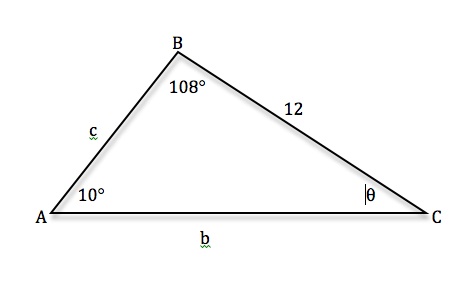




3 answers
Fri Jul 22, 2016 1:08 PM
Post by Peter Ke on June 22, 2016
This is just a curious question. For SSA, you could have no solution, 1 solution, and 2 solution. If the value of sin(theta) is in between -1 and 1 it will have 2 solution. If the value of sin(theta) is less than -1 or greater than 1 there is no solution. Then what value of sin(theta) will produce 1 solution because you did not show that in the video?
1 answer
Thu Jun 5, 2014 11:46 AM
Post by Charlie Jiang on June 1, 2014
Isn't the law of sines this:
a/SinA = b/SinB = c/SinC ?
1 answer
Tue Mar 11, 2014 3:55 PM
Post by Heather Magnuson on March 6, 2014
There are not any examples of a situation with only one solution. Would this happen when the triangle is a perfect right triangle?
1 answer
Tue Aug 13, 2013 5:12 PM
Post by Taylor Wright on July 19, 2013
How do you determine which angle for SSA is going to be the one which could have 2 values?
1 answer
Sun Apr 28, 2013 11:18 AM
Post by JANE CONNER on February 19, 2012
If angle A is obtuse, how can you tell that sine B will be less than 1. Is it because sin b is the supplement of sin A?