Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Trigonometry
For more information, please see full course syllabus of Trigonometry
Trigonometry Sine and Cosine Functions
Lecture Description
Sine and cosine functions are mostly used in terms of right triangles. There are special formulas on how to use them and you can master these formulas, by using the Sohcahtoa. Understanding what a Unit Circle is will help clarify where the sine and cosine functions are. Unit Circle is a circle with a radius of one. When you draw an angle θ in a standard position (starting on the positive x-axis), the coordinates of its terminal side on the unit circle are the cosine and the sine of θ. The x-coordinate is cosine and the y-coordinate is sine. In this lecture you'll learn when the function is odd and when it's even and you'll also practice graphing sine and cosine functions.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine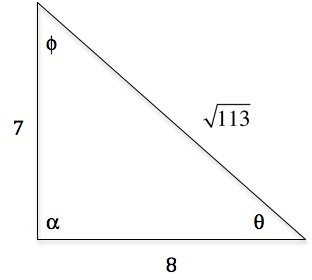





1 answer
Mon Jun 15, 2020 10:24 AM
Post by Victoria Su on June 13, 2020
Hi
For example 4, I understand why they odd/even based on the graph. However, I am not too sure how it works with plugging in the x values for f(x ) or g(x). I tried plugging them in but didn't get the correct answer.
1 answer
Fri Apr 3, 2020 10:03 AM
Post by Cheung Chun Hei on April 1, 2020
I still don't get the part about whether the graph is rotational or mirror symmetry and whether it is odd or even. Can you explain this a bit and thank you so much!
1 answer
Fri Mar 27, 2020 9:55 AM
Post by Yanping Du on March 25, 2020
What happens when a graph is both mirror symmetric and rotationally symmetric? Is that possible?
1 answer
Sun Mar 8, 2020 10:58 AM
Post by susiexuthus on March 7, 2020
When I try to load the extra examples, it says in the bottom right corner "audio source file does not exist". Is it just my computer?
1 answer
Wed Apr 17, 2019 1:35 PM
Post by Christopher Wang on April 16, 2019
I don't understand how you derived the fact that cos pi is -1 and sin pi is 0.
1 answer
Tue Jun 7, 2016 12:31 PM
Post by Bassam Razzaq on June 5, 2016
In example 2, how can the graph has value of x-coordinate more than 2pi. I thought 2pi was the last point as also seen in unit circle.
1 answer
Mon Nov 2, 2015 2:18 PM
Post by Peter Ke on October 30, 2015
In example 4, I DON'T get how g(x) is odd. I thought it was even.
3 answers
Wed Nov 11, 2015 9:23 AM
Post by Peter Ke on October 30, 2015
For example 3 shouldn't Sin = 4/5 be 3/5? Because 3 is the opposite and 4 is adjacent.
1 answer
Thu Feb 19, 2015 3:36 PM
Post by patrick guerin on February 17, 2015
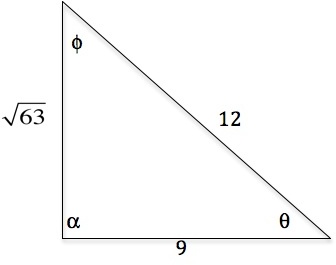
On practice question 2 it said the sin of theta was sqroot of 63 over 12 when i thought it was 9 over 12. Could you check it out please. Thanks.
1 answer
Fri Dec 19, 2014 9:31 AM
Post by katrina williams on December 17, 2014
In the second to last practice problem what amount does n represent? I was able to draw the original graph but got lost by how far to move it over.
1 answer
Mon Aug 4, 2014 7:48 PM
Post by patrick guerin on July 16, 2014
You placed a theta in the triangle that you created when you were defining sine cosine. Could you give me a small explanation on what theta is?
1 answer
Tue Jun 17, 2014 12:08 PM
Post by Austin Cunningham on June 9, 2014
How come at around 8:00, he says that x^2 is the same thing as f(x)?
3 answers
Thu Jun 5, 2014 11:43 AM
Post by Govind Balaji Srinivasa Raghavan on May 29, 2014
I dont know why. But all videos pause after sometime. Then it restarts again instead of continue. Also I cant skip to other part of video. Suppose tonight I watch half of video and go to sleep. Tomorrow morning, I have to watch from first, if i click on the play-head from where I should see, It automatically restarts.
1 answer
Tue Mar 4, 2014 5:00 PM
Post by Damien O Byrne on February 28, 2014
Does sin cos and tan formulas only apply to right angled triangles
1 answer
Wed Jan 22, 2014 3:04 PM
Post by Carroll Fields on January 16, 2014
In extra example I, at 3:30, why is the 'sine, cosine, and tangent of the right angle (alpha) , 1,0 , and undefined?And why for sine is it sin pi/2 ?
1 answer
Mon Oct 21, 2013 7:15 PM
Post by yannick Haberkorn on October 12, 2013
i have to congratulate you Dr.william murray because i actually really feel i am in a learning environment and it feels great . Much thanks
1 answer
Tue Apr 16, 2013 8:35 PM
Post by Dr. William Murray on January 27, 2013
Hi Emily,
Good question. As Jacob says in his post above, it's because we know that the graph of f(x-c) is like the graph of f(x), but shifted c units to the right. But you have to have the negative sign in there for this to work, so when we have f(x+(something)), we write it as f(x-(-something)). Then it's clear that the shift is (-something) units to the right, that is, (something) units to the left.
It's also worth reading Jacob's answer above -- same basic idea, but sometimes having a different person's phrasing helps.
Thanks for taking trigonometry!
Will Murray
1 answer
Tue Apr 16, 2013 8:34 PM
Post by Emily Engle on January 27, 2013
At 28:10 Why do you change Sin (x+ Pi/2) to Sin (x-(- Pi/2)) ?
1 answer
Fri Aug 31, 2012 5:45 PM
Post by Andraa Cram on June 25, 2012
@ 21:18, why, when going in the negative direction while graphing for sine (in red), does he draw the graph as (-Pi/2,-1) instead of (-Pi/2,1)? I'm very confused by this.
1 answer
Sun Jan 27, 2013 4:18 PM
Post by Lourdes Johnson on June 3, 2012
Why does the lecture restart around a quarter in?
1 answer
Sun Jan 27, 2013 4:16 PM
Post by Callistus Elue on May 23, 2012
the lecture reverts to the beginning almost as soon as it starts
1 answer
Sun Jan 27, 2013 4:12 PM
Post by Jacob Burley on April 25, 2011
At 26:33 Professor Murray gave the algebraic equation for a graph that has a constant which was f(x-c). Our equation sin(x+pi/2) has a positive where the negative is in the original equation. In order to get the correct sign there we must change the + sign into two - signs because two negatives make a positive.
I know I'm not the greatest at explaining things but hopefully this helps a little bit.
1 answer
Sun Jan 27, 2013 4:10 PM
Post by Shannon Bryington on February 28, 2011
At 27:40 on the video: Why was x + pi over 2 changed to x - neg pi over 2?
1 answer
Sun Jan 27, 2013 3:59 PM
Post by Santhini Dheenathayalan on January 19, 2011
Great!