Trigonometry Pythagorean Identity

We are learning about the Pythagorean identity, what we are going to try now is to start with the Pythagorean identity and prove the Pythagorean theorem.0000

Remember what we did in the earlier example, was we started with the Pythagorean theorem and we proved the Pythagorean identity.0009

The point of this is to show that you can get from one to the other or from the other back to the first one.0015

And so that the two factor are equivalent even though one seems like geometric fact and one seems like a trigonometric fact.0022

Let us do that, remember that the Pythagorean identity says that sin²(x) + cos²(x) = 1.0033

The Pythagorean theorem, that is the theorem about right triangles, so let me set up a right triangle here.0045

I will label the sides a, b, and c, that is Pythagorean identity, now I’m going to use my SOHCAHTOA.0055

SOHCAHTOA tells us that if we have an angle here, I will this angle (x) in the corner here, the sin(x) is equal to the opposite/hypotenuse.0065

The opposite side here is b/c, the cos(x) is equal to a/c, the adjacent/hypotenuse.0090

I’m going to plug those in the Pythagorean identity because remember we are allowed to use the Pythagorean identity here.0105

That says the sin²(x) + cos²(x) = 1, if I plug those in I will get b/c² + a/c²= 1.0112

Now I’m just going to do a little bit of algebraic manipulation b²/c² + a²/c² is equal to 1.0127

I’m going to multiply both sides there by c² and that will clear my denominators on the left I got b² + a ² and on the right I have c².0137

If I switch around the two terms here a² + b² is equal to c².0151

That is the very familiar equation we approved the Pythagorean theorem.0160

We started out with the Pythagorean identity from trigonometry and we ended up proving the Pythagorean theorem from geometry a² + b²=c².0177

It is just a matter of writing down the right angle in one corner of the triangle and then working through the algebra, you end up with the Pythagorean theorem from geometry.0190

That shows that the Pythagorean identity and the Pythagorean theorem really are equivalent to each other.0200

You ought to be able to start with either one and prove the other one.0206

Let us try one more example, we are given that sin(theta) is -5/13, and (theta) is in the third quadrant and we want to find cos(theta).0000

Let me try graphing out what that might be.0010

Ok (theta) is in the third quadrant, that is down here and so its sum angle down there, I do not know exactly where it is but I will draw it down there.0022

What I’m given is that sin(theta) is -5/13 and I want to find cos(theta).0035

Well I have this Pythagorean identity that says sin²(theta) + cos²(theta) = 1.0040

I will plug in sin(theta ) that is -5/13² + cos²(theta) =10051

-5/13 when you square, the negative goes away so we get 25/169 + cos²(theta) is equal to0062

Well I’m going to have to subtract the 25/169 so I will write 1 as 169/169 then I will subtract 25/169 from both sides.0074

I got cos²(theta) is 144/169, then if I take the square root of both sides to solve for cos(theta), I get cos(theta) is equal to + or – square root of 144 is 12, square root of 169 is 13.0087

I know the my cos(theta) is equal to either positive or negative 12/13.0110

That is all I can get from the Pythagorean identity because it only told me what cos²(theta) is, I can not figure out from that whether cos(theta) is positive or negative.0116

But the problem gave us a little extra information, it says that (theta) is in the quadrant.0127

Knowing that (theta) is in the third quadrant, I looked down there and I remember that cos is equal to the x coordinate of my angle.0133

Cos is the x coordinate, remember all students take calculus, down there in the third quadrant tan are positive but nothing else is positive.0145

That means that cos is not positive, it is negative.0158

The cos(theta) is equal to -12/13 and must be the negative value because it is down in the third quadrant, that is where the x coordinate is negative.0162

The key to this problem is remembering the Pythagorean identity, sin²(theta) + cos²(theta)=1.0181

Then you plug the value you are given into the Pythagorean identity and you try to solve for cos(theta).0189

Once you work through the arithmetic you get the value for cos(theta) but you do not know if it is positive or negative.0197

Then you go over and look whether what quadrant the angle is in, it is in the quadrant and then you either remember the all students take calculus.0203

That tells you the plus or minus on the different functions or you just remember that in the third quadrant the x values are negative so the cos value has to be negative.0212

Either way you end up with cos(theta) is equal to -12/13.0222

That is the end of our set on the Pythagorean identity, this is www.educator.com.0229

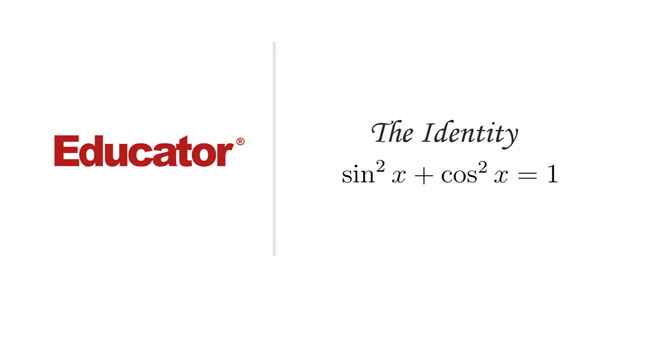
Hello, this is the trigonometry lectures for educator.com and today we're going to learn about probably the single most important identity in all trigonometry which is the Pythagorean identity.0000

It says that sin²x + cos²x = 1.0011

This is known as the Pythagorean identity.0017

It takes its name from the Pythagorean theorem which you probably already heard of.0019

The Pythagorean theorem says that if you have a right triangle, very important that one of the angles be a right angle, then the side lengths satisfy a² + b² = c².0024

You probably heard that already.0040

The new fact for trigonometry class is that sin²x + cos²x = 1.0042

What we're going to learn is we work through the exercises for these lectures.0050

Is if these are really two different sides of the same coin, you should think of this as being sort of facts that come out of each other.0055

In fact, we're going to use each one of these facts to prove the other one.0064

These are really equivalent to each other.0069

Let's go ahead and start doing that.0071

In our first example, we are going to start with the Pythagorean theorem, remember that's a² + b² = c².0074

We're going to try to prove the Pythagorean identity sin²x + cos²x = 1.0084

The way we'll do that is let x be an angle.0095

Let's draw x on the unit circle.0107

The reason I'm drawing it on the unit circle is because remember the definition of sine and cosine is the x and y coordinates of that angle.0112

If we draw x on the unit circle, the hypotenuse has length 1 and the x-coordinate of that point, remember, is the cos(x), and the y-coordinate is the sin(x).0124

Now, what we have here is a right triangle and we're allowed to use the Pythagorean theorem, we're given that and we're going to use that and try to prove the Pythagorean identity.0148

The Pythagorean theorem says that in a right triangle, by the Pythagorean theorem...0160

Let me draw my right triangle a little bigger, there's x, there's 1, this is cosx, this is sinx.0174

By the Pythagorean theorem, one side squared, let me write that first of all as cosine x squared plus the other side squared is equal to 1².0186

That's the length of the hypotenuse.0205

If we just do a little semantic cleaning up here, 1², of course, is just 1, cosine x squared, the common notation for that is cos²x + sin²x = 1.0207

We just derived an equation, and look this is the Pythagorean identity.0228

What we've done is we started by assuming the Pythagorean theorem and then we used the Pythagorean theorem to derive the Pythagorean identity.0246

Let's see an application of that in the next example.0256

We're given that θ is an angle whose cosine is 0.47, and θ is in the fourth quadrant.0260

We have to find sinθ.0266

Let me draw θ, θ is somewhere down there in the fourth quadrant.0272

I don't know exactly where it is but θ looks like that.0279

Here is what I know, by the Pythagorean identity, sin²θ + cos²θ = 1.0284

I'm going to fill in the one that I know, cosθ, cosθ is 0.47.0295

This is 0.47² = 1 + sin²θ.0302

Now, 0.47, that's not something I can easily find the square of, so I'll do that on my calculator.0310

0.47² = 0.2209, so that's +0.2209, sin²θ +0.2209 = 1, sin²θ = 1 - 0.2209, which is 0.7791.0317

Sinθ, if we take the square root of both sides, sinθ is equal to plus or minus the square root of 0.7791, which is approximately equal to 0.8827.0360

Now, it's plus or minus because I know that sine squared is this positive number, but I don't know whether this sine is a positive or negative.0382

We're given more information in the problem, θ is in the fourth quadrant.0390

Remember, sine is the y-coordinate, so the sine in the fourth quadrant is going to be negative because the y-coordinate is negative.0395

Because θ is in quadrant 4, sinθ is going to be negative, so we take the negative value, sinθ is approximately equal to -0.8827.0414

The whole key to doing this problem was to start with the Pythagorean identity sin²θ + cos²θ = 1.0446

Once you're given sine or cosine, you could plug those in and figure out the other one except that you can't figure out whether they're positive or negative.0457

Their identity doesn't tell you that so we had to get this little extra information about θ being in the fourth quadrant, that totals that the sinθ is negative and we were able to figure out that it was -0.8827.0463

Let's try another example of that.0480

We're going to verify a trigonometric identity.0483

This is a very common problem in trigonometry classes as you'll be given some kind of identity involving the trigonometric functions and you have to verify it.0486

For this one, what I want to do is start with the right hand side.0496

I'm going to label this RHS.0503

RHS stands for right-hand side.0504

The right-hand side here is equal to sinθ/(1 - cosθ).0510

Now, I'm going to do a little trick here which is very common when you have something plus something in the denominator, or something minus something in the denominator.0519

The trick is to multiply the conjugate of that thing.0529

Here I have 1 - cosθ in the denominator, I'm going to multiply by 1 + cosθ, and then, of course, I have to multiply the numerator by the same thing, 1 + cosθ).0533

The reason you do that, this is really an algebraic trick so you probably have learned about this in the algebra lectures.0547

The reason you do that is you want to take advantage of this formula, (a + b) × (a - b².0554

That's often the way of simplifying things using that algebraic formula.0566

What we get here in the numerator is (sinθ) × (1 + cosθ), in the denominator, using this (a² + b²) formula, we get (1 - cos²θ).0570

Now, let's remember the Pythagorean identity.0586

Pythagorean identity says sin²θ + cos²θ = 1.0590

That means 1 - cos²θ = sin²θ.0596

We can substitute that in into our work here, sinθ×(1 + cosθ).0603

The denominator, by the Pythagorean identity, turns into sin²θ.0613

We get some cancellation going on, the sine in the numerator cancels with one of the sines in the denominator leaving us just with (1 + cosθ)/sinθ in the denominator.0623

That's the same as the left-hand side that we started with.0639

We started with the right-hand side and we're able to work it all the way down and end up with the left-hand side verifying the trigonometric identity.0644

There were sort of two key steps there.0654

One was in looking at the denominator and recognizing that it was a good candidate to invoke this algebraic trick where you multiply by the conjugate.0657

If you have (a + b), you multiply by (a - b).0669

If you have (a - b), you multiply by (a + b).0672

Either way, you get to invoke this identity.0674

Here, we had (a - b), we multiplied by (a + b) and then we got to invoke the identity and get something nice on the bottom.0679

The second trick there was to remember the Pythagorean identity and notice that (1 - cos²θ) converts into sin²θ.0686

Once we did that, it was pretty to simplify it down to the left-hand side of the original identity.0697

We'll try some more examples later.0703