Connecting...
This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Algebra 2
For more information, please see full course syllabus of Algebra 2
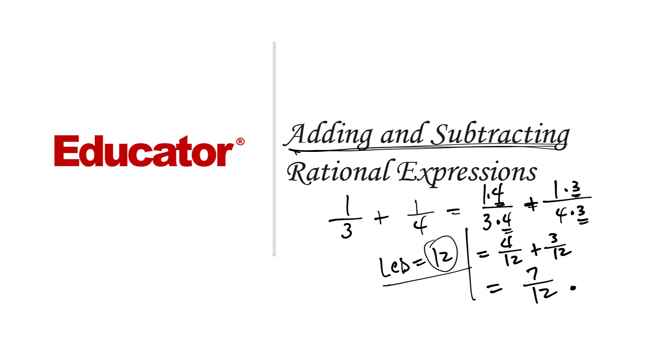
Algebra 2 Adding and Subtracting Rational Expressions
Lecture Description
Adding and subtracting rational expressions are more complicated than multiplying and dividing, but we are going to apply the same techniques we used to add and subtract fractions involving numbers. The first step is to find the least common multiple (LCM), which is obtained by factoring the polynomials. Then adjust the numerator and denominator of each fraction so that its denominator is the LCM. Finally, add or subtract the numerators and simplify the result. When dealing with complex fractions, add or subtract the fractions in the numerator and the denominator separately. Then simplify the resulting expression. This is more complicated than dealing with complex fractions that do not involve polynomials.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture









































 Carleen Eaton
Carleen Eaton Grant Fraser
Grant Fraser
 Answer Engine
Answer Engine



0 answers
Post by Hong Yang on June 21, 2020
ydougissucas? pls answer
0 answers
Post by Hong Yang on June 21, 2020
yugissucass?
0 answers
Post by Dr Carleen Eaton on May 24, 2012
Julius, if I understand the question, at 15:00, it is easier to factor out the 2. Otherwise your LCD is (x-3)(x 1)(2x-6). No reason it shouldn't work but it is a little more complicated and to get the most simplified form you'll have to do a difficult factoring of a cubic equation at the end.
1 answer
Last reply by: Hong Yang
Sun Jun 21, 2020 10:06 AM
Post by julius mogyorossy on May 21, 2012
Dr. Eaton, in the example you gave us I did not factor out the two first, I just factored it, did I do it the wrong way, it seems so, when I substitute 1 in for x it seems I get a different solution than you, but it really seems the way I did it should work 2, if you must factor out the 2 first, why so please?