Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Algebra 2
For more information, please see full course syllabus of Algebra 2
Algebra 2 Hyperbolas
Lecture Description
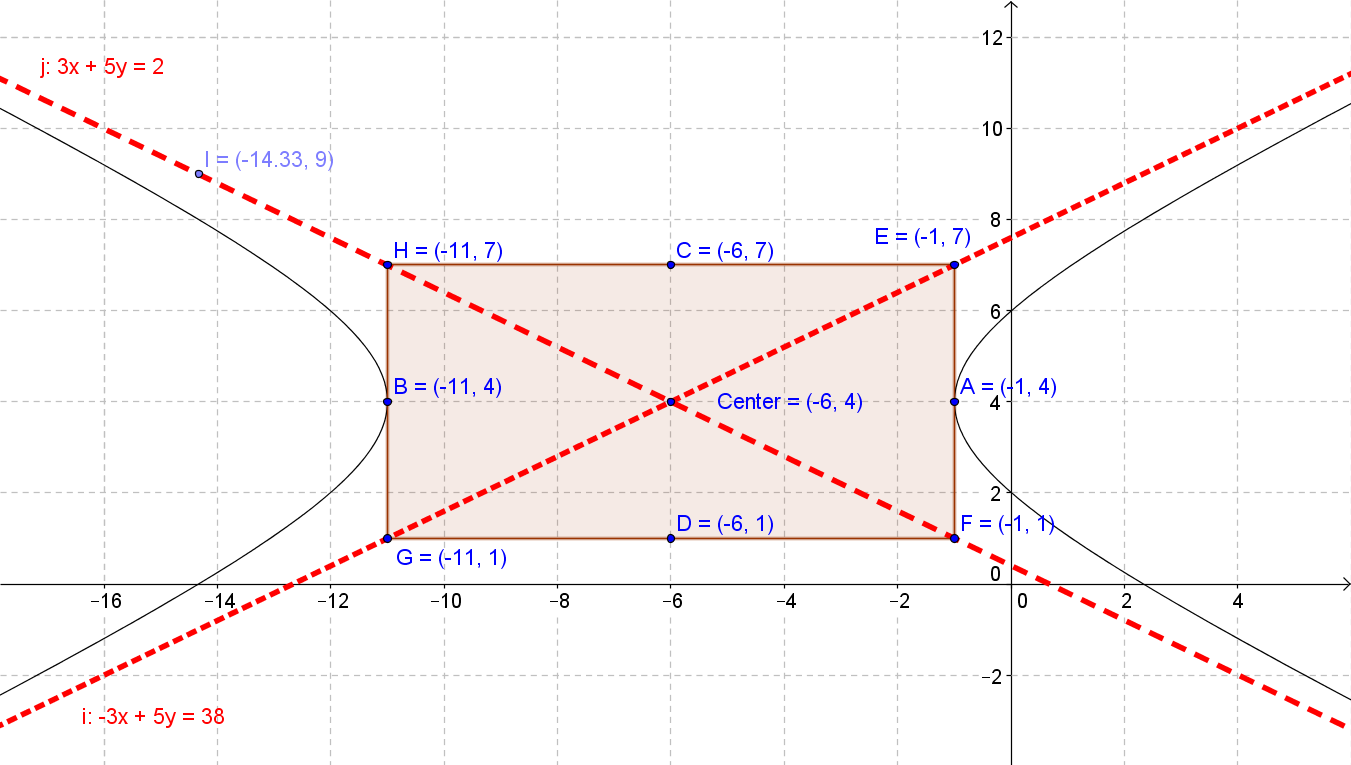
Hyperbolas are a bit different in shape than the other conic sections we've learned about. A hyperbola is the set of points in the plane such that the absolute value of the difference of the distances from two fixed points is constant. The two points are the foci of the hyperbola. A hyperbola has two axes of symmetry, called the transverse axis and the conjugate axis, which intersect, at the center of the hyperbola. The transverse axis intersects the vertices of the hyperbola. In this lecture you'll learn about the standard form of the hyperbola and how to put an equation in standard form by completing the square.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture









































 Carleen Eaton
Carleen Eaton Grant Fraser
Grant Fraser
 Answer Engine
Answer Engine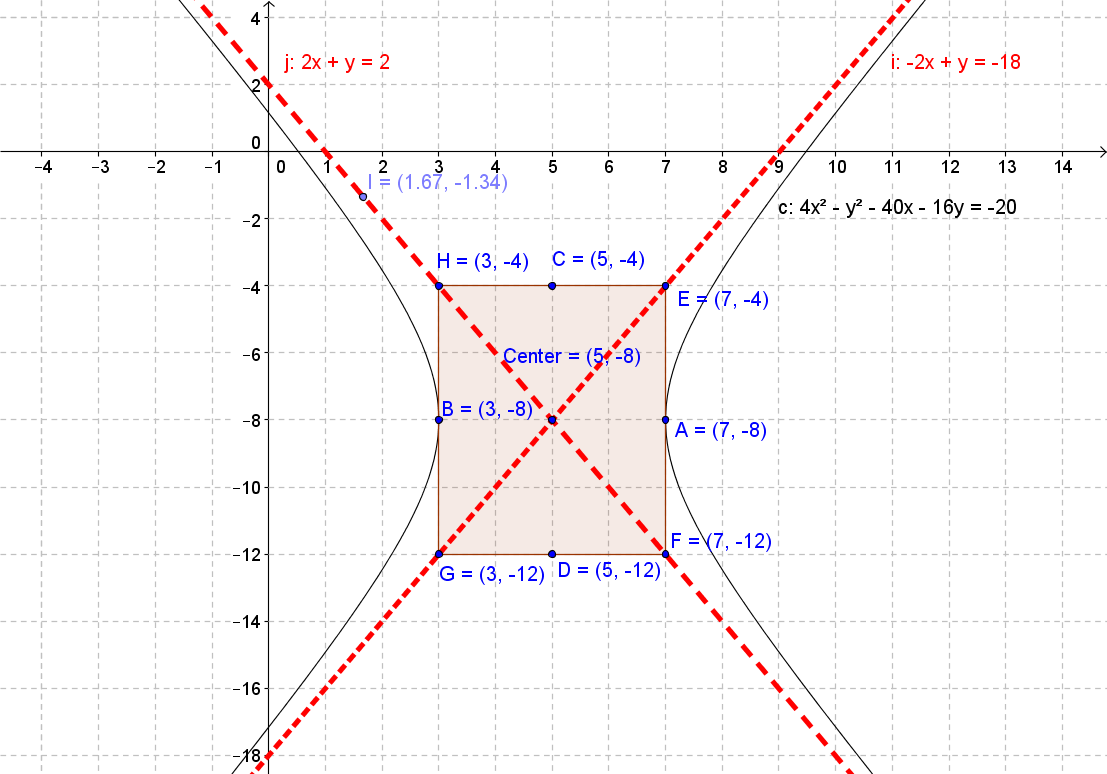




1 answer
Sun Apr 8, 2018 2:39 PM
Post by Shiden Yemane on March 21, 2018
In example 3, when you were completing the square for the x terms, shouldn't the the (b^2) over 4 be (-2)^2 over 4, instead of 2^2 over 4? Let me know if I'm wrong.
3 answers
Last reply by: Chessdongdong
Mon Jun 1, 2020 1:25 PM
Post by Kenneth Montfort on March 6, 2013
So, you said in the lecture on ellipses, that a^2 was the larger term, it seems here that b^2 is the larger term...is that another clue about how to tell which formula you are using?