Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Congruent and Similar Solids
Lecture Description
In this lesson, we are going to go over congruent and similar solids. Whenever we have two solids that are either similar or congruent, there is a scale factor. A scale factor is just the ratio that compares the two solids; it is the ratio of the corresponding measures (it has to be corresponding). For congruent solids, these have to be true: the corresponding angles are congruent; the corresponding edges are congruent; the areas of the faces are congruent; and the volumes have to be congruent. And congruent solids have the same size and same shape. This lesson also covers similar solids.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine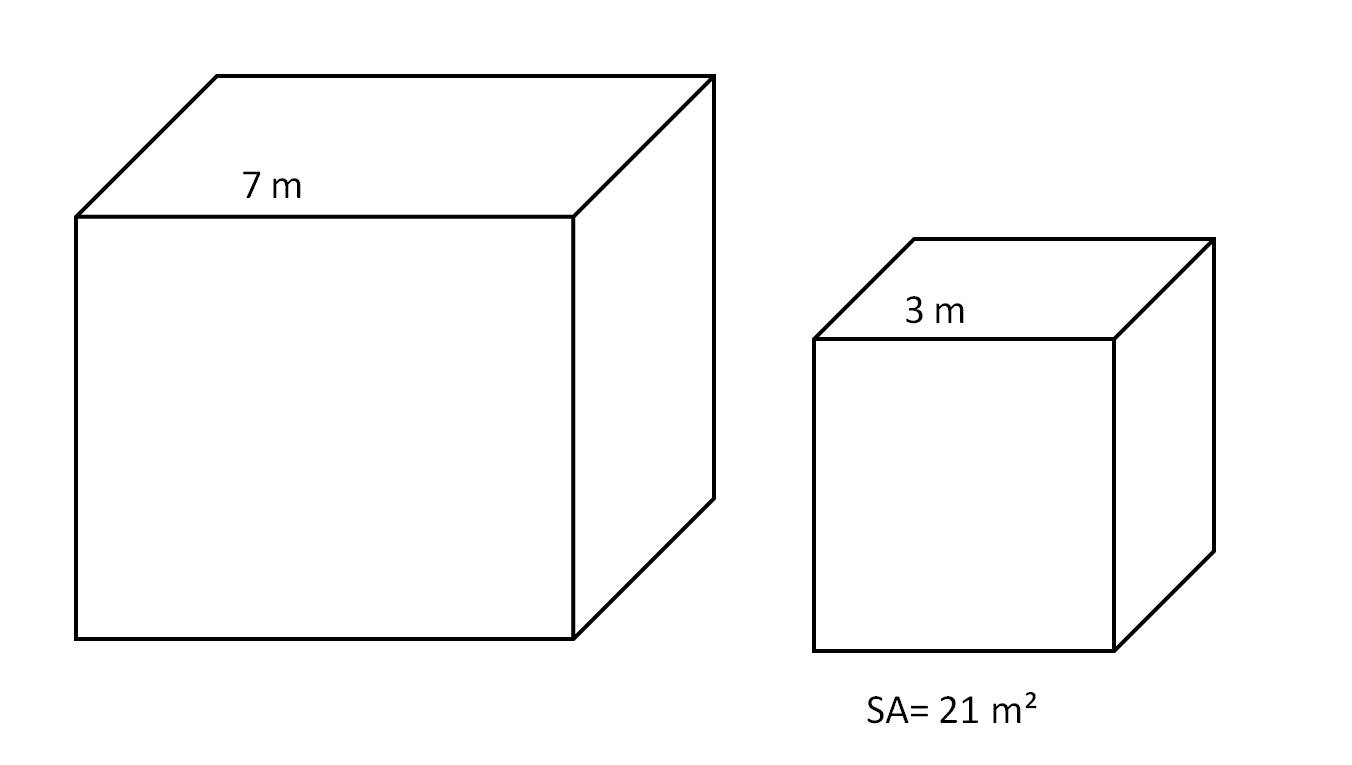




0 answers
Post by chengpingru on November 13, 2019
On extra example 1; second shape: you solved and got the statement that the two cylinders have the same height. However from what I know, a cylinder, hence a circular prism with the same width all the way up. If it is already given that both of the solids have the same diameter/radius. Why do you need to solve for the hight if it is going to be the same width all the way up? I think that all you really need to know is that the height is the same, instead of knowing the exact hight of the cylinder. Otherwise, they will not be congruent or simular.
0 answers
Post by Shahram Ahmadi N. Emran on August 4, 2013
Thanks