Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Rectangles
Lecture Description
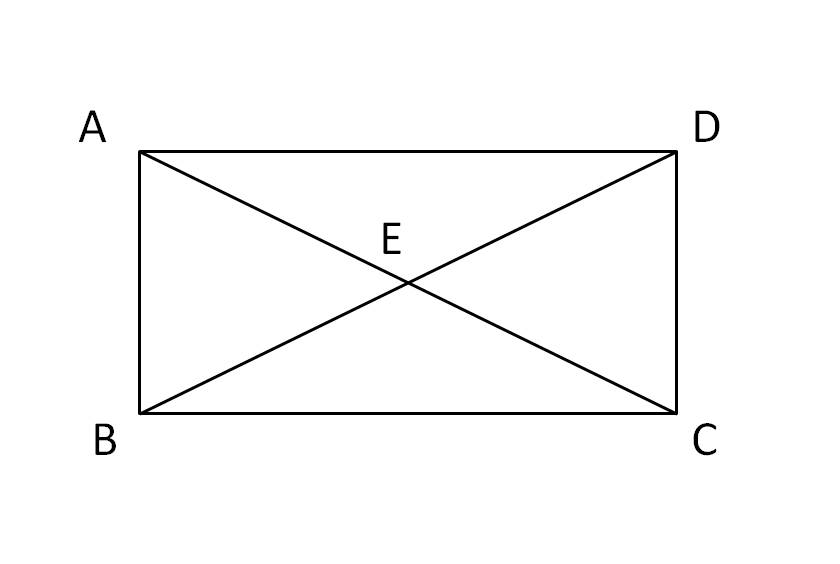
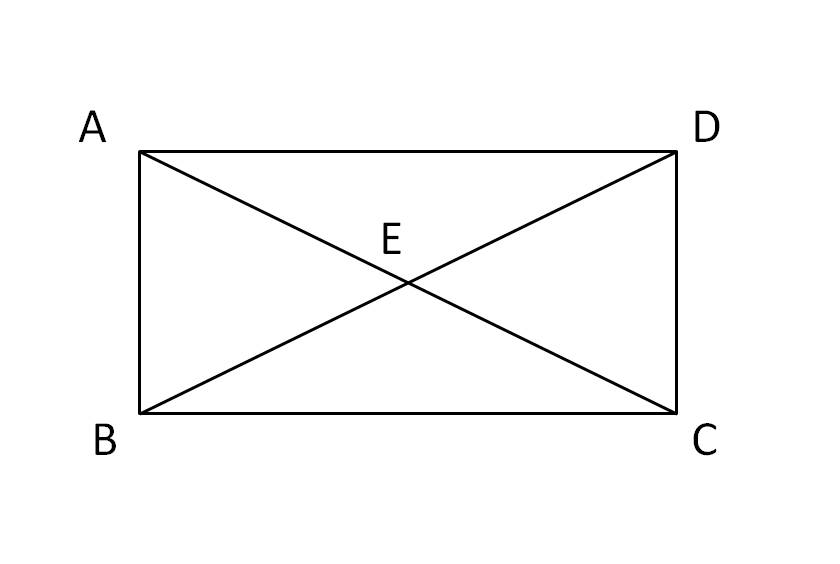
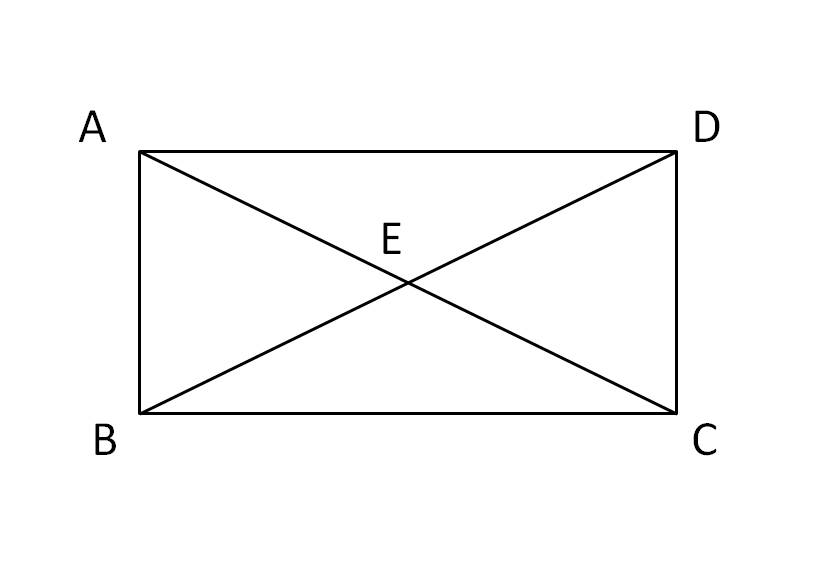
This lesson is on rectangles. A rectangle is a quadrilateral with four right angles; we know that there are only four angles in a rectangle, so all four are right angles. Rectangles are a special type of parallelograms, so it has all the properties of a parallelogram. For example, opposite sides are parallel, and two pairs of opposite sides are congruent, as well as two pairs of opposite angles are congruent. We also know that diagonals bisect each other. Those are all of the properties of a parallelogram. However, rectangles have some more properties than the parallelograms. For example, the diagonals of a rectangle are congruent. You'll learn about some other properties of rectangles in this lesson.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine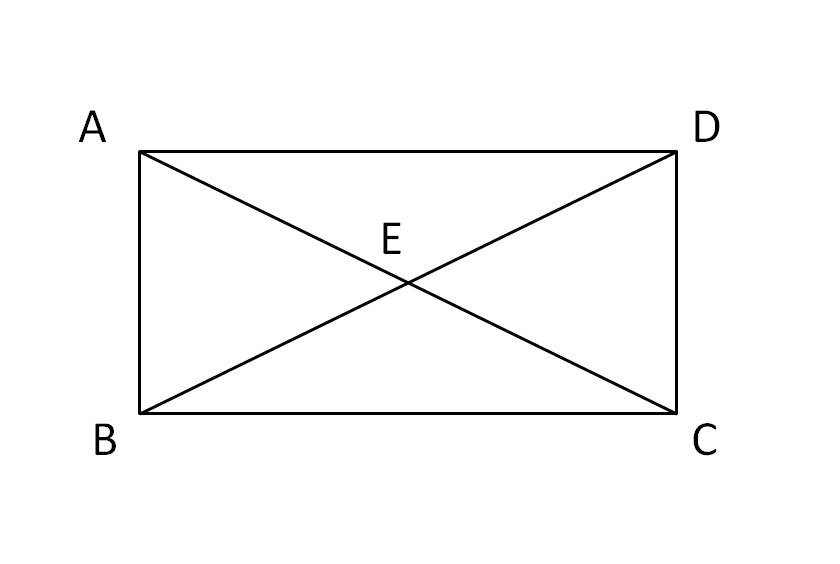




0 answers
Post by Tami Cummins on July 31, 2013
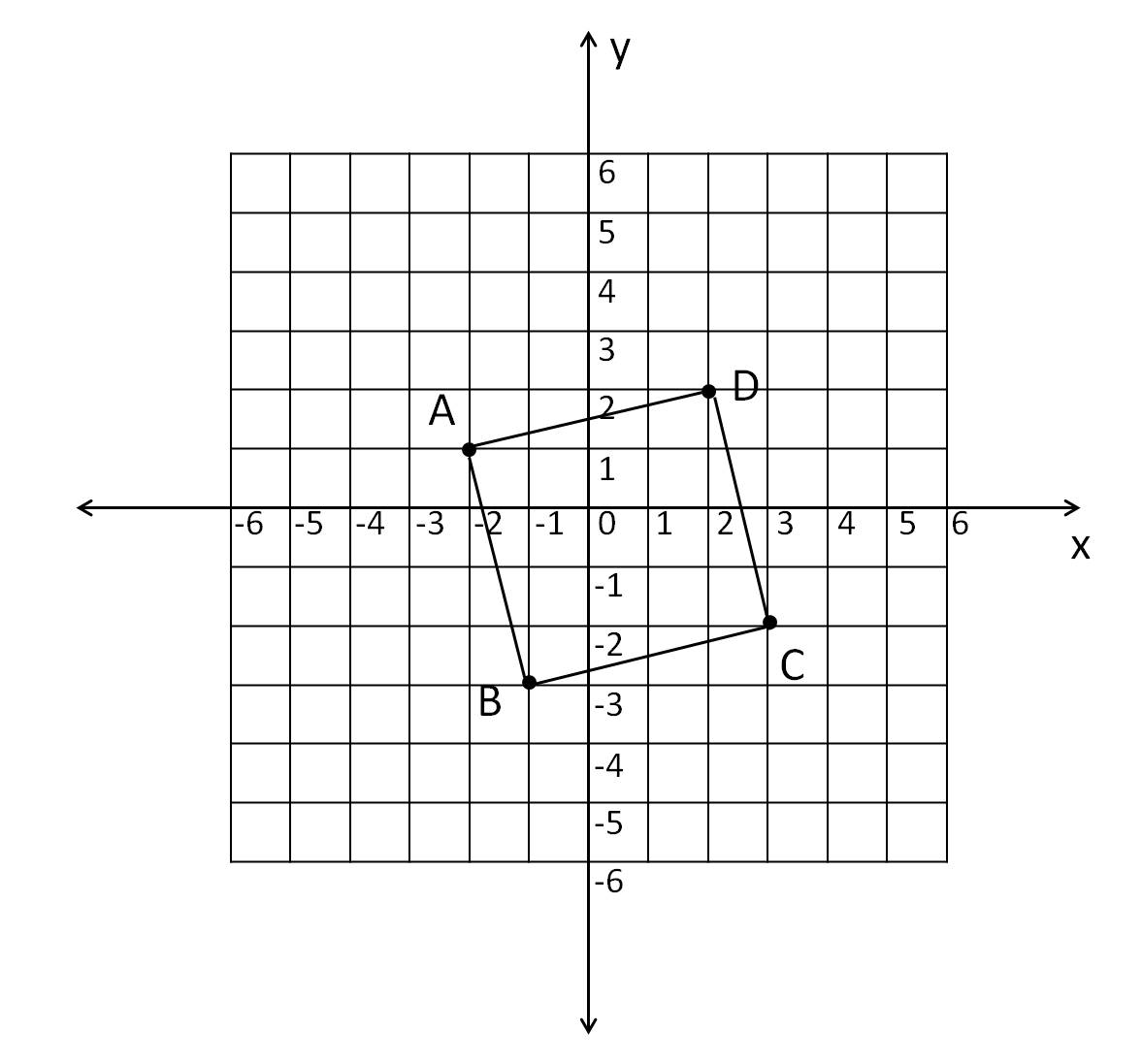
I meant isn't even a parallelogram is it?
3 answers
Thu Jan 2, 2014 3:52 PM
Post by Tami Cummins on July 31, 2013
On example 3 number 3. I thought the definition of parallelogram was that opposite sides are parallel and congruent. If that is true then wouldn't a parallelogram with a right angle have to have 4 right angles and thus be a rectangle. The example you gave is even a parallelogram is it?