Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Law of Cosines
Lecture Description
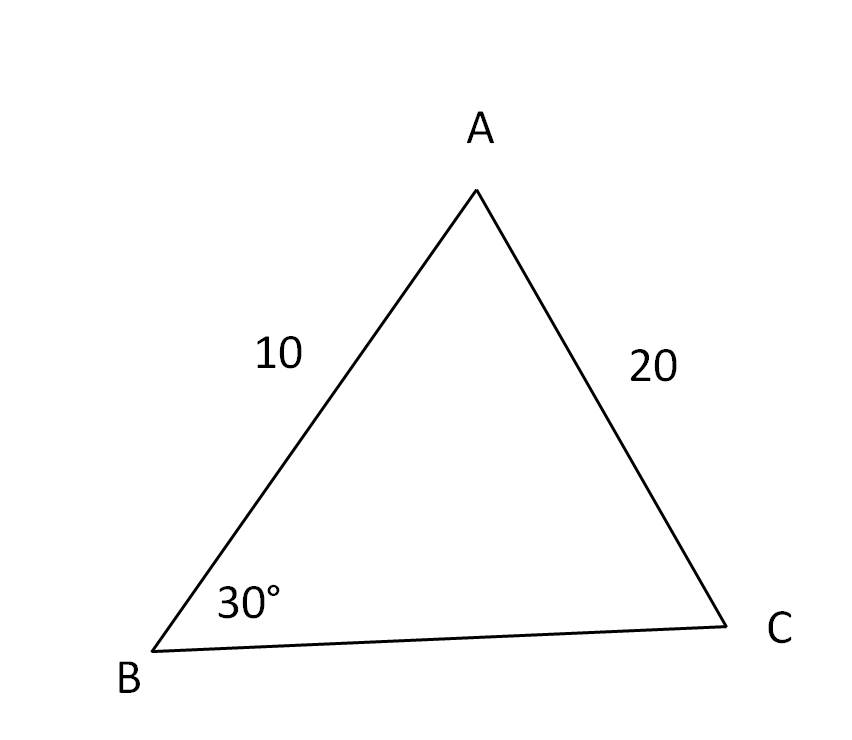
In this lesson we are going to talk about the Law of Cosines. Using Law of Cosines is very similar to when we used Law of Sines. We are using both of those for non-right triangles; it is very important to remember that there are different things that you can use for right triangles, and then there are things that you can use for non-right triangles. The Law of Cosines consists of three different formulas, depending on what you need - what measure you have to find. You'll learn how all these formulas have the same pattern, which will help you remember them easily.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine
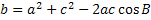
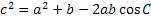




0 answers
Post by Todd McKinney on December 9, 2014
You make it a lot easier to understand.
Thanks
1 answer
Sat Feb 4, 2012 12:48 AM
Post by Sanjay Nenawati on October 27, 2011
a-s-a is does have a angle side relationship, because 180 - a -a= other angle. then you will have a side angle side relationship.