Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Inscribed Angles
Lecture Description
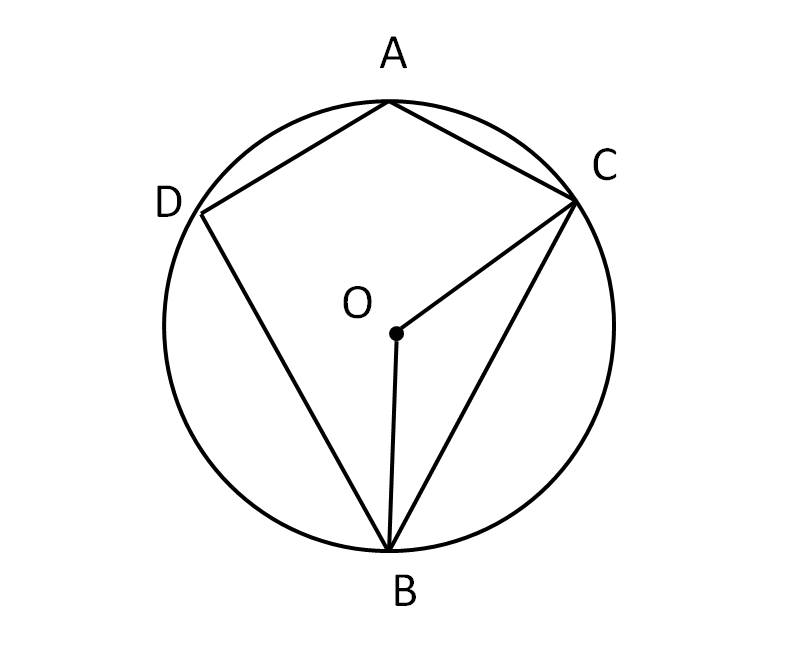
For this lesson, we are going to go over inscribed angles of circles. An inscribed angle is an angle within a circle whose vertex is on the circle. This lesson covers some important inscribed angles theorems. One of them says that the inscribed angle is half the measure of the intercepted arc. You'll also learn about inscribed angles that intercept congruent arcs, and some special cases when the inscribed angle intercepts a semicircle. Angles of a quadrilateral inscribed in a circle have an interesting property, and we use that property when solving many of the geometry problems.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine





1 answer
Last reply by: Briahnna Austin
Wed Apr 20, 2016 2:59 AM
Post by Briahnna Austin on April 20, 2016
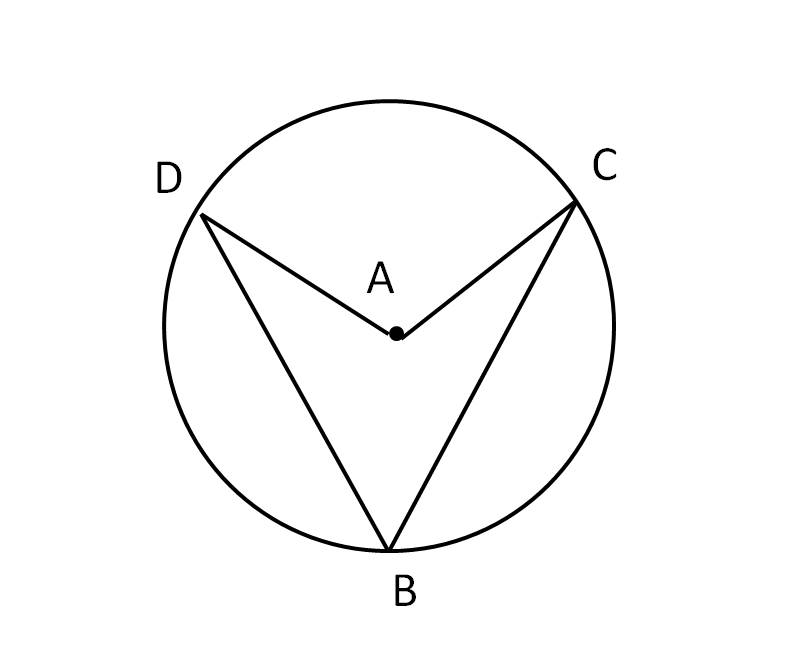
Hello, this video was great, but I had a question about the central angles you listed.
By definition you said the central angle is related to the center. The point is in the middle and the chords extend to the end of the circle-- you listed <CPB, and <APB as central angles and I was wondering why is <APC or <CPA not listed as a central angle, since it follows the qualifications a central angle should have?