Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Proving Triangles Congruent
Lecture Description
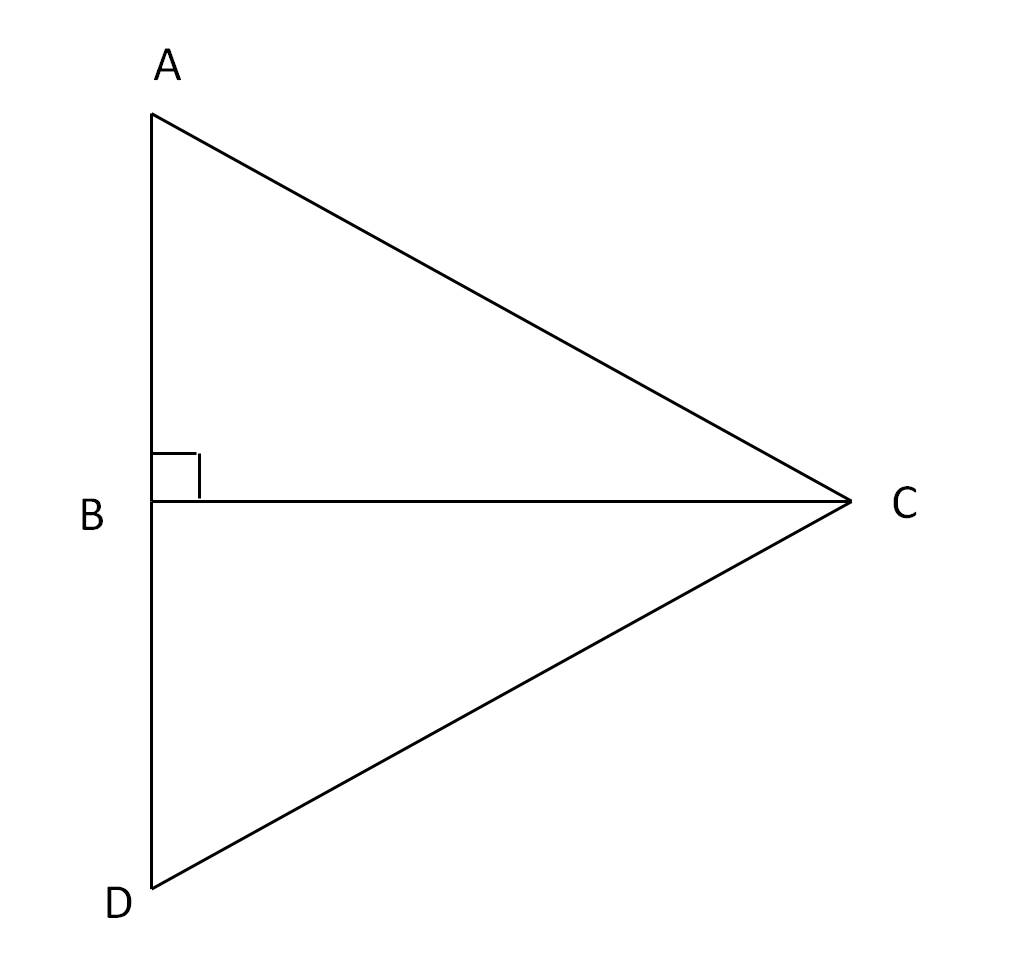
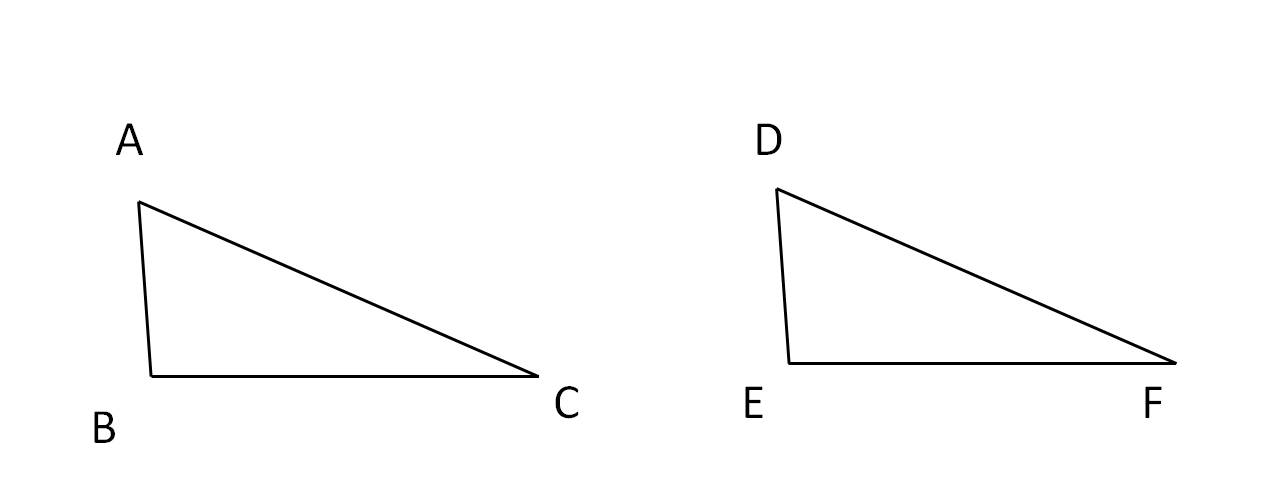
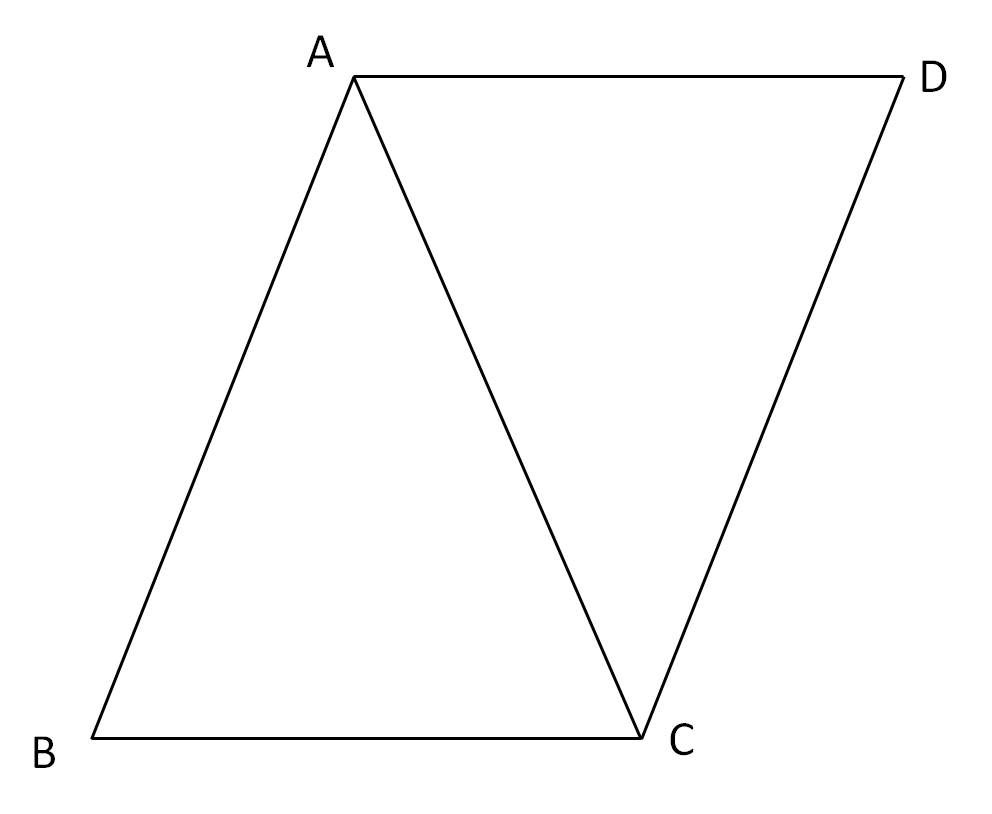
In the previous lesson we talked about the definition of congruent triangles, and how, if we have two triangles, their corresponding parts are going to be congruent. In this lesson we are going to take a closer look at some of the methods we can use to prove that two triangles are congruent. According to the definition of congruent triangles, if all of the corresponding parts of the two triangles are congruent, then the two triangles are congruent. But we don't always have to prove the congruence of all six parts. Instead, we have postulates and theorems that make it easier. In this lesson, you'll learn about the SSS postulate, the SAS postulate, the ASA postulate, and the AAS postulate.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine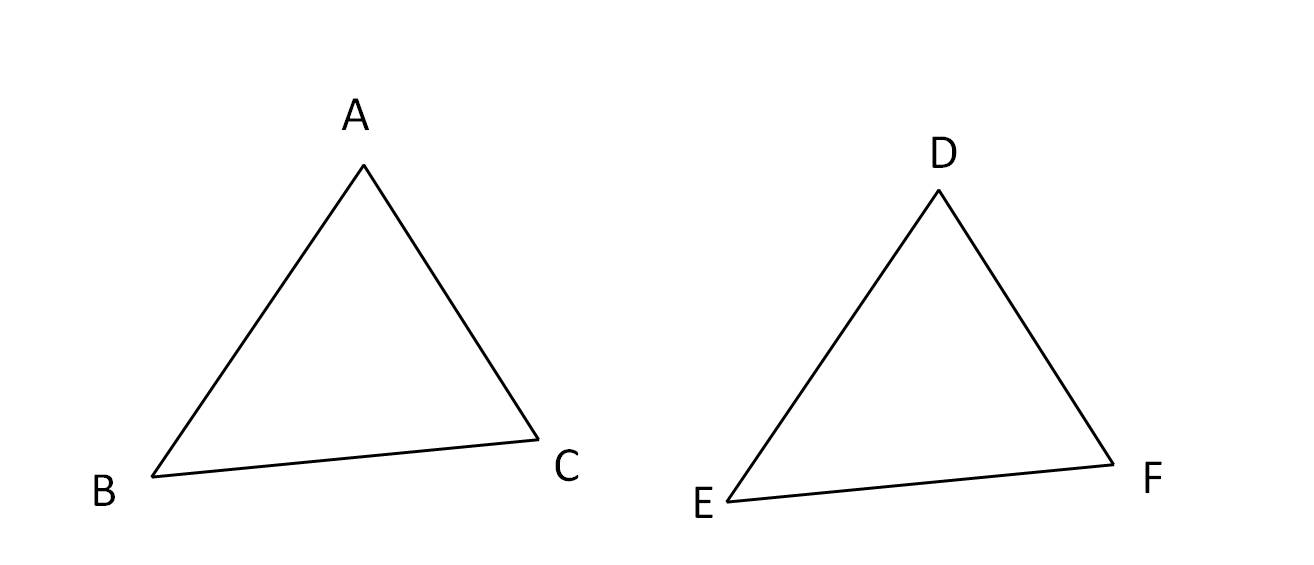




0 answers
Post by Mei Gill on October 21, 2018
Well in class I am learning HL which is hypotenuse length, and are u going to teach this?
2 answers
Last reply by: John Stedge
Wed Jul 25, 2018 9:29 AM
Post by John Stedge on July 25, 2018
The ways of proving triangle congruence should not be considered a postulate it should be considered a theorem because you have to prove the triangles congruence. Otherwise you could have two incongruent triangles and state that they are congruent by say SSS and you would just have to believe me even though they are obviously not congruent.
0 answers
Post by Kristie Ornellas on November 16, 2016
Can we show a proof example of SSS postulate so I can understand that postulate? With the SAS proof I understood that postulate perfectly. So do you think you can show a proof example somehow please.
0 answers
Post by Mohammed Jaweed on August 12, 2015
0 answers
Post by Shahram Ahmadi N. Emran on July 1, 2013
Why the lectures kept stopping in the middle of the slide which is being taught?
0 answers
Post by jeeyeon lim on January 16, 2013
love your examples!!!!!!
1 answer
Last reply by: Shahram Ahmadi N. Emran
Mon Jul 1, 2013 1:13 PM
Post by Nadarajah Vigneswaran on November 17, 2012
Do the vertical angles have to be in the triangle or can they be exterior. For example on your example of the sas postulate if triangles BEC and AED were non existent could you use the exterior angles formed at point E as proof that angle AEB and angle CED.
0 answers
Post by reid brian on February 15, 2012
hey man! no...^
2 answers
Sun Sep 11, 2011 9:14 PM
Post by Sayaka Carpenter on August 22, 2011
for the SAS postulate, in the example you drew, the angle that you used as an example was the top angle, but can it be any of the 3 angles of the triangle? i dont really get the inside triangle part...