Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Geometric Probability
Lecture Description
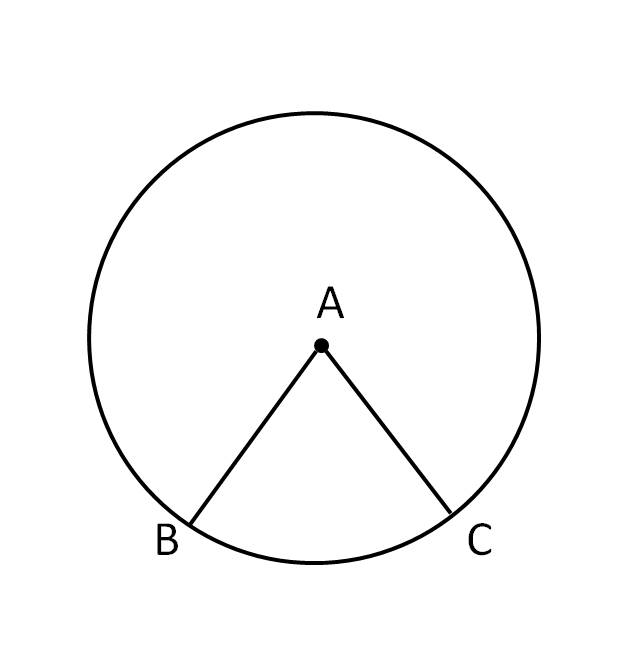
For this lesson, we are going to go over geometric probability. The first thing that we are going to go over is the Length Probability Postulate. This postulate says that if a point on AB is chosen at random and C is between A and B, then the probability that the point is on AC is AC/AB. Besides the length probability postulate, we are also going to go over the area probability postulate. This one deals with a point in a region chosen at random. This lesson also covers the area of a sector of a circle.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine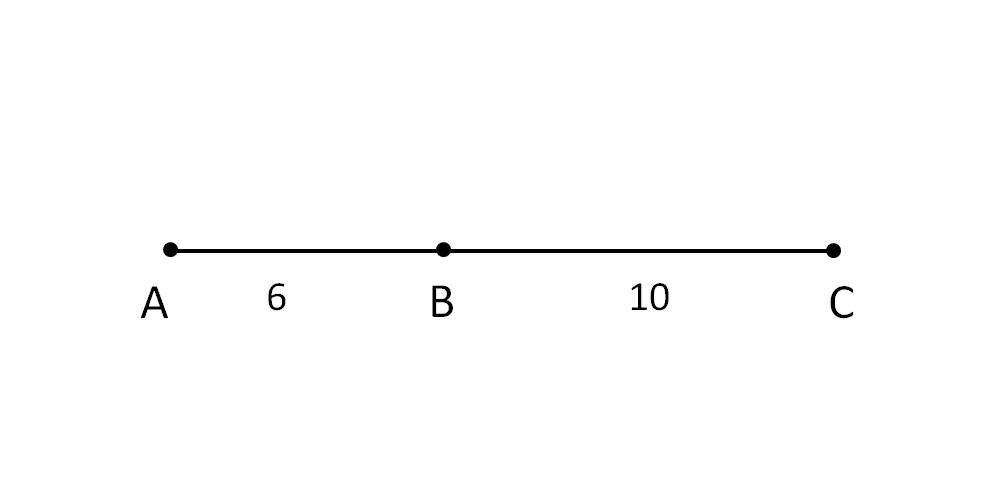




0 answers
Post by Jamal Salim on October 27, 2015
in Example 2 you can find the probability direct using 72/360 = 1/5 because (Pi)r2 will cancel each other
2 answers
Last reply by: Jamal Salim
Tue Oct 27, 2015 8:57 PM
Post by Mirza Baig on December 6, 2013
I think the example 3 where you find the side of a square and you said that it was 10 radical 2 but in my opinion i think that's wrong because we know that squares sides and hypotenuse are same because of Pythagorean theorem
and so add 10+10 = 20,20 is the side of a square so the area of a square would be 400cm^2.