Connecting...

This is a quick preview of the lesson. For full access, please Log In or Sign up.
For more information, please see full course syllabus of Geometry
For more information, please see full course syllabus of Geometry
Geometry Volume of Pyramids and Cones
Lecture Description
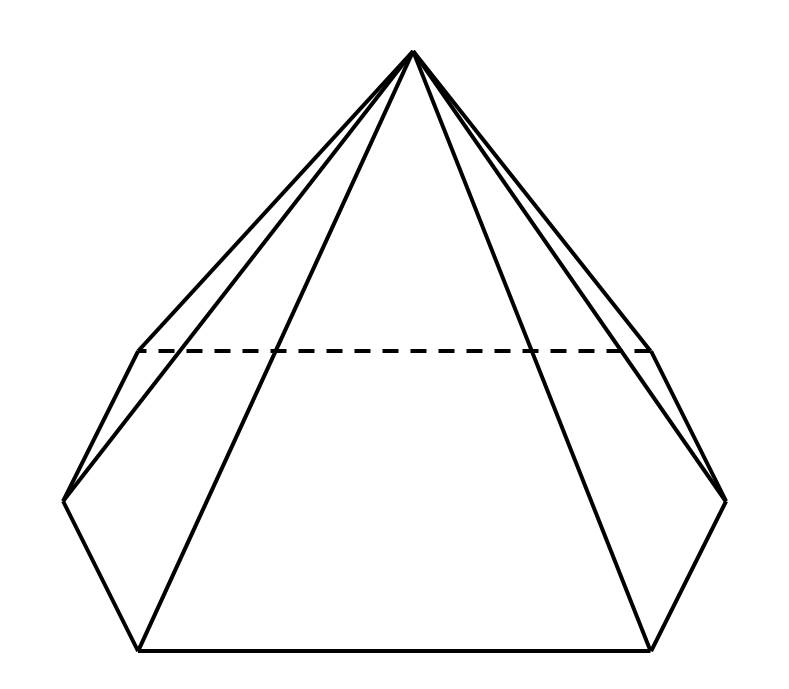
In this lesson, we are going to go over volume of cones. Remember that volume is how much space is inside the actual solid. The volume of a cone is one third of area of base times the height. Since the base is the circle, we'll use the area of a circle here. For a pyramid, it's actually exactly the same formula, one third of area of a base times the height. The base is a polygon, so if it is a square we'll use the area of a square. You'll practice solving problems that involve finding the volume of different solids.
Bookmark & Share
Embed
Share this knowledge with your friends!
Copy & Paste this embed code into your website’s HTML
Please ensure that your website editor is in text mode when you paste the code.(In Wordpress, the mode button is on the top right corner.)
×
Since this lesson is not free, only the preview will appear on your website.
- - Allow users to view the embedded video in full-size.
Next Lecture
Previous Lecture










































 Answer Engine
Answer Engine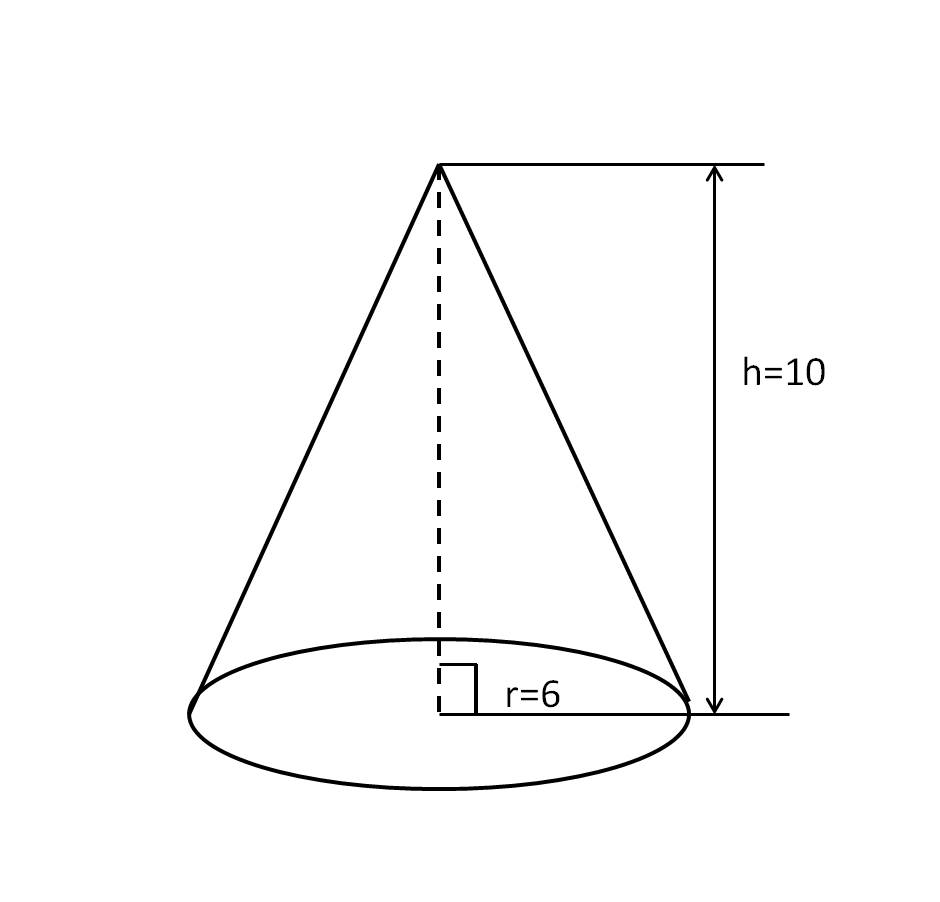
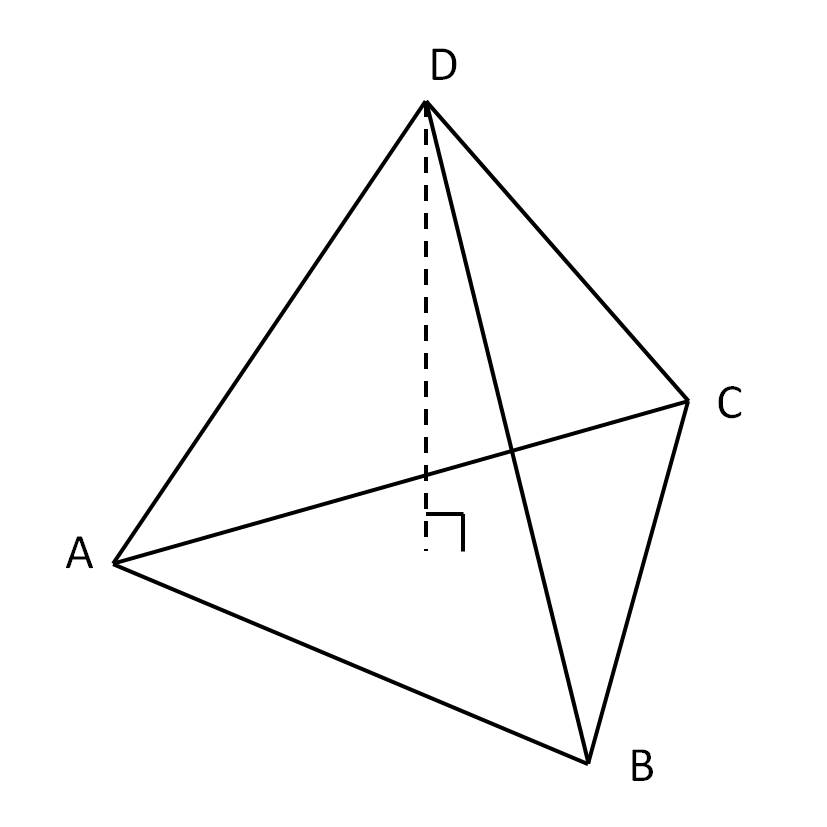 Find the volume of the tetrahedron, the base ABC is a equilateral traingle, the length of each side is 8, the height of the tetrahedron is 9.
Find the volume of the tetrahedron, the base ABC is a equilateral traingle, the length of each side is 8, the height of the tetrahedron is 9. 



0 answers
Post by Christopher Wang on April 9, 2019
Isn't a cone just a circular pyramid?
0 answers
Post by Professor Pyo on August 2, 2013
*NOTICE* THERE IS A MISTAKE IN EXAMPLE IV
The height is NOT 10, it is 7.1! It would not be 8.7 because the height has to be the measure that is going straight down to be perpendicular to the base. In this example, I meant for 8.7 to be what's called the "slant height," where it is the height of the triangle face (notice how it's slanted) and not the height of the actual pyramid.
In the case you are given a "slant height" instead of the actual height, you would have to use pythagorean theorem (refer to the red triangle in the pyramid that I drew). The "slant height" of 8.7 would be the hypotenuse, 5 would be one side (since it's half the side of the square), and the actual height would be the unknown side.
h^2 + 5^2 = 8.7^2
h = 7.1
SO the correct volume of the octahedron:
V = (1/3)(10)(10)(7.1) (2)
V = 473.3 cm^3
Hope this clears up the mistake! I apologize for the confusion!! (Sigh, wish I was perfect and never made any mistakes.) =/
1 answer
Fri Aug 2, 2013 3:22 AM
Post by Norman Cervantes on June 29, 2013
I think you made a mistake in the fourth example. you said v=(1/3)(10)(10)(10) the last ten being the height which should be 8.7 instead am i right?